Algebra Equations

Read the following SAT test question and then select the correct answer. 

Use the same method for all SAT math questions.  Read the question carefully, identify the bottom line, and assess your options for solving the problem.  Choose the most efficient way to solve the problem, and attack it!  Finally, loop back to make sure that you solved for the bottom line.

If  and , then t exceeds s by

Bottom line:  You need to know how much t exceeds s.  So the question you are asked is really, “How much bigger is t than s?”  Your bottom line is t – s.

Assess your options:  Normally you would simplify s before plugging the s value into the equation for t, then find the difference between t and s.  However, doing this will give you some ugly fractions and take a lot of time.  Instead, try starting with your bottom line and plugging in everything that you know.

Attack the problem:  Start with the t – s and plug in the equations for both of these:

Then plug in s one more time:

This looks terribly ugly, but keep calm and use the order of operations.  You always multiply or divide before you add or subtract, so your first job is to distribute the half within the brackets by multiplying it by every number within the first set of parentheses:

Look at the new equation that you have.  How many things cancel out when you start subtracting the second group of numbers from the first?  Almost everything!  You are left with a single fraction:



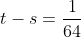
Loop Back:  You solved for t - s, your bottom line, so you are ready to look at the answer choices.

(A)
(B)
(C)
(D)
(E)

The correct answer is (E).

On sat.collegeboard.org, 41% of the responses were correct.

For more help with math questions, visit www.myknowsys.com!